Keep it in the Family (with Pythagorean Triples)

As we came in the room, Eric asked us to place a post-it with our name on a voting spectrum he’d drawn on the board, ranging from “Never” to “This morning” under the statement, “The last time I thought about multiplication”.

As we settled in, Eric shared his goals for the meeting. He’s been working on a lesson for students combining some work he’s been doing on area models with a problem that has been consuming him for weeks. He said he was going to skip some of the scaffolding he is building for students so that we can be sure to get to the problem at the heart of the lesson.

---

Eric started us off with a warm-up activity from the website, Which One Doesn’t Belong? Given the four numbers below, we had to come up with one quality that made each number different from the other three numbers.

After a few minutes, we shared what we came up with:

(For more ideas on how to use Which One Doesn’t Belong? in adult ed classes, check out Learning Through Classification, a review on CollectEdNY.org)

---

Area Models in Multiplication

Segueing from the 121 in the WODB square, Eric introduced how to use an area model to visual (and calculate) 11².

He drew an 11 by 11 square, then broke it up into 4 sections – a 10 by 10 square (100), two 10 by 1 rectangles (20) and a single box (1)

He then asked us to use area models to find 13², 16², and 23².

Haewon’s most closely matched the 11² example.

Rather than always using the 10 by 10 square, Tyler became interested in looking for patterns in using different perfect squares to cover the area. Here are a few examples of his work on the 13 by 13 square.

Then we had a brief discussion of the partial product method for multiplication, as it relates to the area model. The area model allows you to visual the different products in the partial product method.

13

×13

9 —-3×3

30—-10×3

30—-10×3

100—-10×10

169

Then Eric had us look for the connections between the area models and the standard algorithm for multiplying. This would be a rich activity to explore further with students.

One final thing that came up is that the area model/partial products method is particularly effective when you are doing the math in your head. (For a great activity on helping students develop mental math, check out Mental Math to Increase Student Computational Fluency and Number Sense, a review on CollectEdNY.org.)

---

Pythagorean Triples – Part 1

Next Eric connected our work with squares to the Pythagorean Theorem, less a²+b²=c² and more “the area of a square built off the hypotenuse of a right triangle is equal to the sum of the areas of the squares build off the legs”.

 

Then he gave us the following picture:

and the prompt, “Investigate the relationships between the lengths of the sides of the triangles that belong to this set.”

We played around with it by ourselves at first, and then we worked in groups.

After Eric brought us together, he asked us what our initial observations of the triangles were. We said we noticed:

• the hypotenuse minus one equals the longer leg.
• the shorter legs are going up by two.
• they all have 1 even side and 2 odd sides.
• there is a function to find the short leg, long leg, hypotenuse (linear, quadratic, quadratic)

Several of us took the put the lengths in a chart and found some interesting patterns that allowed us to predict other triples that would be part of this set. One of the things we noticed was that the difference in the lengths of the longer legs were increasing by 4. (+8, +12, +16, +20…)

Mark

Tyler

Haewon took a different approach. She noticed that, taken in order of the smaller leg (3 is the first, the 5 is second, the 7 is third and the 9 is fourth), the larger legs can be expressed as 4 times 1, then 4 times 1+2, then 4 times 1+2+3 then 4 times 1+2+3+4.

Haewon also noticed that the largest number she was adding (before multiplying the sum by 4) was always equal to one less than the length of the smaller leg divided by two. For example, in the 9, 40, 41 triangle the 40 can be expressed as 4(1+2+3+4) with 4 being the largest number added. 9 (the smaller leg) minus 1 is 8, 8÷2 is 4. This allowed her to figure out all three sides from any given side.

For example:

• If the smaller side was 21.
• 21-1 is 20. 20/2 is 10.
• 1+2+3+4+5+6+7+8+9+10 is 55.
• 4×55 is 220, so the longer leg is 220
• The hypotenuse is 221, or one more than the longer leg.

Solange noted that we’ve seen that pattern (1, 1+2, 1+2+3, 1+2+3+4…) at other CAMI meetings (especially when we looked at Toothpick Patterns – January 2015)

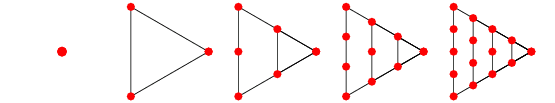
It’s the triangular numbers!

(What are they doing here in this family of Pythagorean Triples?)

It was interesting to see that both approaches – the chart and the visual – involved the number 4. What is the relationship between the change in the differences of the longer sides and as the number being multiplied by the triangular numbers? Where does that 4 comes from?

---

 Pythagorean Triples – Part 2 (Extension)

Then Eric gave us the following extension:

Time ran out before we had a chance to discuss the extension, but here are a few of the categories my group came up with:Another question Eric posed to us as he walked from group to group was, “See if you can categorize these triples into different families”.

• Triples with a difference of 2 between side b and side c
• Triples with a difference of 8 between side b and side c
• Triples with a difference of 9 between side b and side c
• Triples with an even shorter leg
• Triples with leg with a length that is a multiple of 3

Haewon and I were looking at the triples that had different differences between the longer leg and the hypotenuse when time ran out.

---

Some questions remain:

• What are the different families of triples with particular differences between the longer leg and the hypotenuse? (at today’s meeting we saw families with differences of 1, 2, 8 and 9) Is there a pattern in the “difference families”?
• How can we figure out any of the three sides if given one side for all of the different “difference families”?
• Is there one rule to bind them when it comes to figuring out any Pythagorean triple?
• Does every Pythagorean triple fit into one of these “difference families”?
• Why are the triangular numbers found in that first family of Pythagorean Triples – where there was a difference of 1 between the longer leg and the hypotenuse? Are the triangular numbers found in any of the other Pythagorean triple families?
• Why is the difference between b and c remain constant within a family (1, 2, 8, 9…)? It seems like the gap should be larger as the triangles get bigger and bigger?
• Is there a size limit on the right triangles in any of the “difference families”? Are there infinite triples within each “difference family”?

---

Teaching Resources

Area Models

---

In attendance: Solange, Cynthia, Simone, Brian, Haewon, Mark, Chaim, Linda, Eric, Tyler

Programs represented: Brooklyn Public Library, BMCC, Literacy Assistance Center, Lehman College Adult Learning Center, CUNY Start, CUNY Adult Literacy PD Team, NYCDOE, Bard Prison Initiative, Fifth Avenue Committee

Location: BMCC, 25 Broadway, 8th floor, Manhattan

Respectfully submitted by Mark

Save