In April, along with some other CAMI members, Jane and Solange went to the National Council of Teachers of Mathematics (NCTM) annual meeting in San Francisco. In this meeting, they shared some of their favorite games, puzzles and problems from different workshops.
We started with the game Which Number is Closest? from Building Mathematical Thinking Through Number Games, by Linda Dacey and Jayne Bamford Lynch. We played a variation of the game where we each rolled a ten-sided die and then wrote down each number in the box of our choice.
The person closest to the goal number on the left got a point for each number. For example, in the first line, I was 848 points higher than the target number of 100.
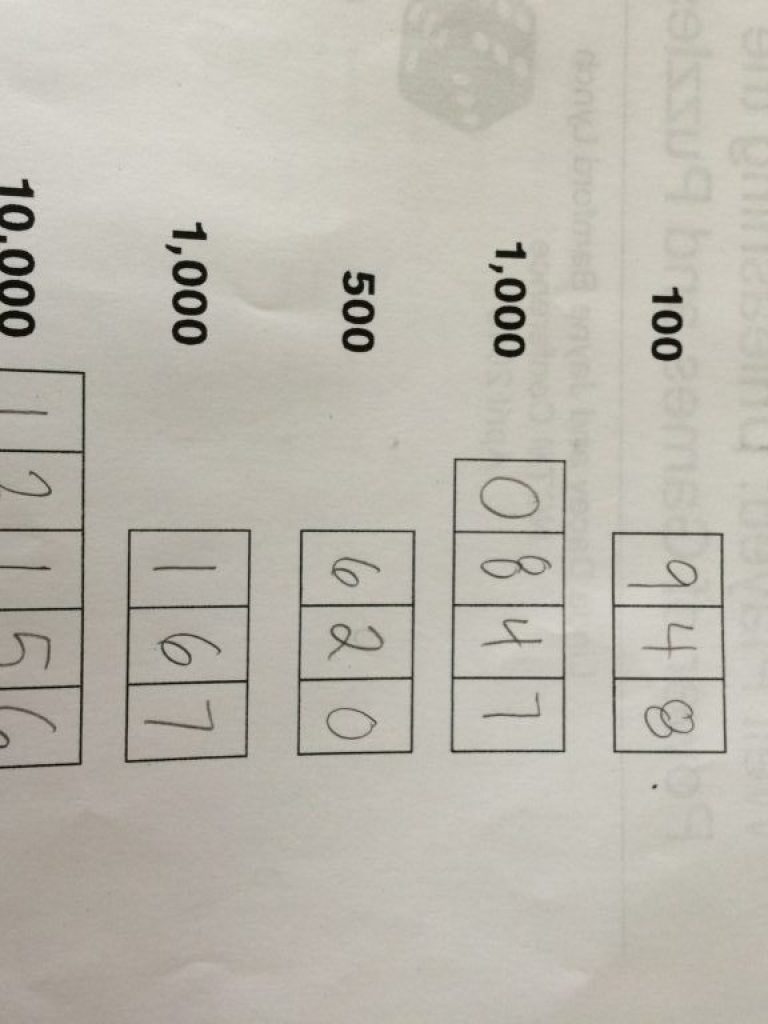
In the original version of this game, cards with 18 numbers (1-9 twice) are shuffled and turned over one at a time. This version would allow students to predict what numbers are left. Solange created the modification with a 10-sided die to complicate the game, but you might use the original version to first introduce it. Instructions and the game board are available in the Well Played handout.
We then looked at some problems from One’s Good But More is Better, by Michael Serra, who has written books on using patty paper for teaching geometry. Solange related that Serra talked about the two main elements of a good math problem: multiple solutions or multiple strategies (or both). We started with a “mental jogging” activity in which Solange asked the group, “If you were stranded on a desert island and you could bring one person, who would you want?” The goal of this kind of question is to encourage students to think creatively and use their background knowledge and life experiences in the math classroom, rather than waiting for the teacher to show them a procedure.
The following question was of a similar purpose:

Then we looked a puzzle:

We came up with solutions to some but not all of these conditions. There was some agreement that we wouldn’t use this puzzle with our students, at least not without modification.
Solange moved us along with the question, “How many line segments do you see in this figure?”

We did the counting individually and then shared our methods for counting the line segments.


Then Solange gave us an extension for the train: What would the next figure look like? How many line segments would be in the nth figure? Etc. One thing to note is that there are different ways to count line segments. For example, Fig. 1 would be made with 16 toothpicks, but there is a way to count 28 different line segments in Fig. 1 (I think!).
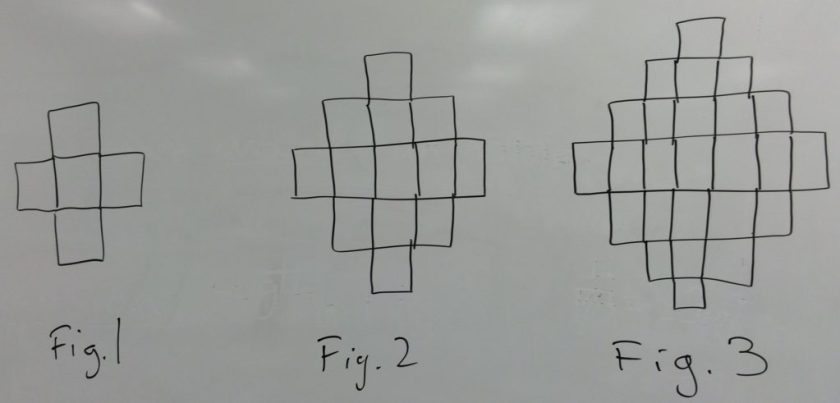
The materials from Michael Serra’s workshop are available in the One’s Good but More’s Better.
At this point, Linda brought up the recent movie The Man Who Knew Infinity about the mathematician Ramanujan and told us a story from the movie. Another mathematician is late to meet Ramanujan and says the taxi’s number was inauspicious (1729) and Ramanujan responded immediately, “I disagree. It’s the smallest number that can be expressed as the sum of two cubes in two different ways.” Do you see what he meant?
Jane then shared a couple activities from Mathalicious. She started with the activity Downside Up: Is There an Upside to Negative Feelings?, which uses clips from Inside Out and line graphs to explore happiness and depression through signed numbers and absolute value. We also looked at You’re So Fined, which uses clips from John Oliver’s show on the problem of municipal fines (which was also highlighted by a federal investigation in Ferguson, Missouri) and the terrible ramifications on people in poverty. The lesson uses starting amount and rate of change to look at fines and probation fees for people who aren’t able to pay immediately. Other acitivities from Mathalicious include triangulating cell phone towers stealing second base (pythagorean theorem – FREE lesson). You need a subscription to Mathalicious in order to use most of their lesson plans and materials, but a personal membership is only $5/month. Jane swears by it.
And finally, Linda and I played the fantastic game, Win-A-Row, which involves addition of signed numbers. It’s a two-player game. One at a time, one person plays negative numbers and the other plays positive numbers. The goal is to make sums that are either positive or negative, depending on which numbers you’re playing. We only played one game but started to see that there could be interesting strategies. It would definitely capture some students’ competitive streaks.

Win-A-Row and ton of other materials are available in the Making Middle School Math Come Alive 2013 handouts.
NCTM’s page for resources from the conference: https://nctm.confex.com/nctm/2016AM/webprogram/start.html
Thank you so much to Jane and Solange for sharing these wonderful resources from NCTM!


